Performance

(shout into the tube)
Ahhhhhhhhhhhhhhhhhhhhhhhhhhhhhh!

You think you got rid of it, but it’s still there…
She said,
“You think you got rid of it, but it’s still there.
I’m telling you, it’s gonna come back, as soon as this spring.”

I like donuts. I really like donuts. When I like something, I stroll around the town to find the best of the best. This year I was really into donuts. It’s not too close by, but I would go to Greenpoint, to Peter Pan Bakery. Really lite it, cheap, creamy, and good fresh baked donuts, you know. In Manhattan, I liked a pistachio donut at Balthazar. But really, the best shop is Donut Plant. That Donut Plant… One thing you have to be careful about Donut Plant is, they close when they sell out all the donuts they have. Isn’t it really hard to prioritize donuts before leaving the work? But since they may sell out, after work, you have to rush there. Because, you know, otherwise… I had learned this through a hard lesson.

(shout into the rube)
Ahhhhhhhhhhhhhhhhhhhhhhhhhhhhh!

I met seven psychics this summer. I met seven of them, and they all said the same thing. They all said things that other people don’t know about me, and then they all said the same thing afterward. It’s creepy.

“You think you got rid of it, but your cancer is still there, it’s going to come back, as soon as this spring if you keep living the way you did.”

Certain things you just know, only you know, like you already know…

The second psychic I met was the worst one, you know the type that has overly nice hair and in a very expensive coat. She had this very rich fox fur thing, even though in the summer. She had an SUV and she… I mean, how would I trust this person, to tell me the truth? Besides she is earning a lot of money anyhow.

You should be careful. You know, when I was setting this installation up, I had to be up high to rig and I was using the ladder. And then…

(balance on the tube and pointing empty area to the audience)
Um, hey, even though I hung that from high, it’s still dangling kind of low. So if you have to go to the other side of the room right now, just be careful. You have to take time, slow down, and be careful, watch out.

(swing one of the hung glasses)
This might hit your head.

Anyway, when I was setting this up on the ladder, I noticed every ladder I had during that week of installation said,
“Do not step on the top step. It’s dangerous.”
What happens if you step on the top of the ladder?

You know, that Donut Plant is on Grand street. I was just walking on Grand street to go to Donut Plant. That was one summer evening. Right then, what happened was, I was so focused on the donuts that I didn’t notice this psychic coming by. Usually I’m pretty good at spotting, by the time I met three psychics, I have learned what kind of person that is. On seeing who they are, I turn around and run away, you know. Somehow that time, I was so focused on going to Donut Plant, that I forgot to watch somebody coming from the side. And she caught me in the middle of the street. She took me, she took me by the wrist, and pulled me to the other side of the street. Then told me the same thing,
“It’s gonna come back this spring.”

The thing that I don’t really get is that she somehow had one hand on my wrist, and the other she had a bag full of donuts. For fifteen minutes, she kept my wrist. By the time she let me go and I crossed the street, Donut Plant just closed in front of me. Sold out. And she… She had a bag full of donuts.

(rotate the table while chanting Hannya Shingyo into the microphone)
Maybe, maybe if I keep moving around, I could get away from all those. All of them but also what they say.

When I was small, I had this recurring dream that I was a strawberry. I got eaten. I got eaten by myself. It was an interesting journey. Maybe because I think I was into encyclopedias back then and just watched their biology pages. It was a kind of philosophical trip. I’m starting to lose redness of me and those seeds all around my skin, and I’m starting to lose the whole strawberry characteristics. And getting attacked by all the acid, peptone, pepsin, and meeting all the intestines… that was a lot of fun. And then at the end of it, I always meet this priest called Hemorrhoid. Hemorrhoid told me,
“Look, do you know if you are now inside or outside of your body? I am inside and outside of the body at the same time.”
I guess a human body is like a tube, you know. They are just the same thing.

“You think you got rid of it, but it’s still there.
I’m telling you it’s gonna come back, as soon as this spring.”

How would you eat this donut inside out?

(hand one donut to an audience)
How would you eat this donut inside out?
The question is how you can go inside without taking anything apart and go all the way in the middle and eat from inside out.

Can I be inside and outside at the same time? Hemorrhoid said,
“It’s very lonely, to be inside and outside at the same time.”
Maybe so.

This is the equation of Lorenz Attractor. Lorenz attractor is basically one of the strange attractors. Strange attractor is a mathematical concept you can find in some simple graphs. You find these two equations and you get a simple graph. The graph seems to move very fast, this happened in the 80s or something; they started to put these differential equations into a computing system to see what it draws. And what happened was… Okay, so somehow these graphs seem to move around certain points, lines, or planes. In this case, Lorenz Attractor, they go around two points back and forth, circulating around these points numbers of times, but nobody knows exactly when it moves to the next phase.

And now there is one thing that I wanna teach you about today. It is about people in general. People in this world.

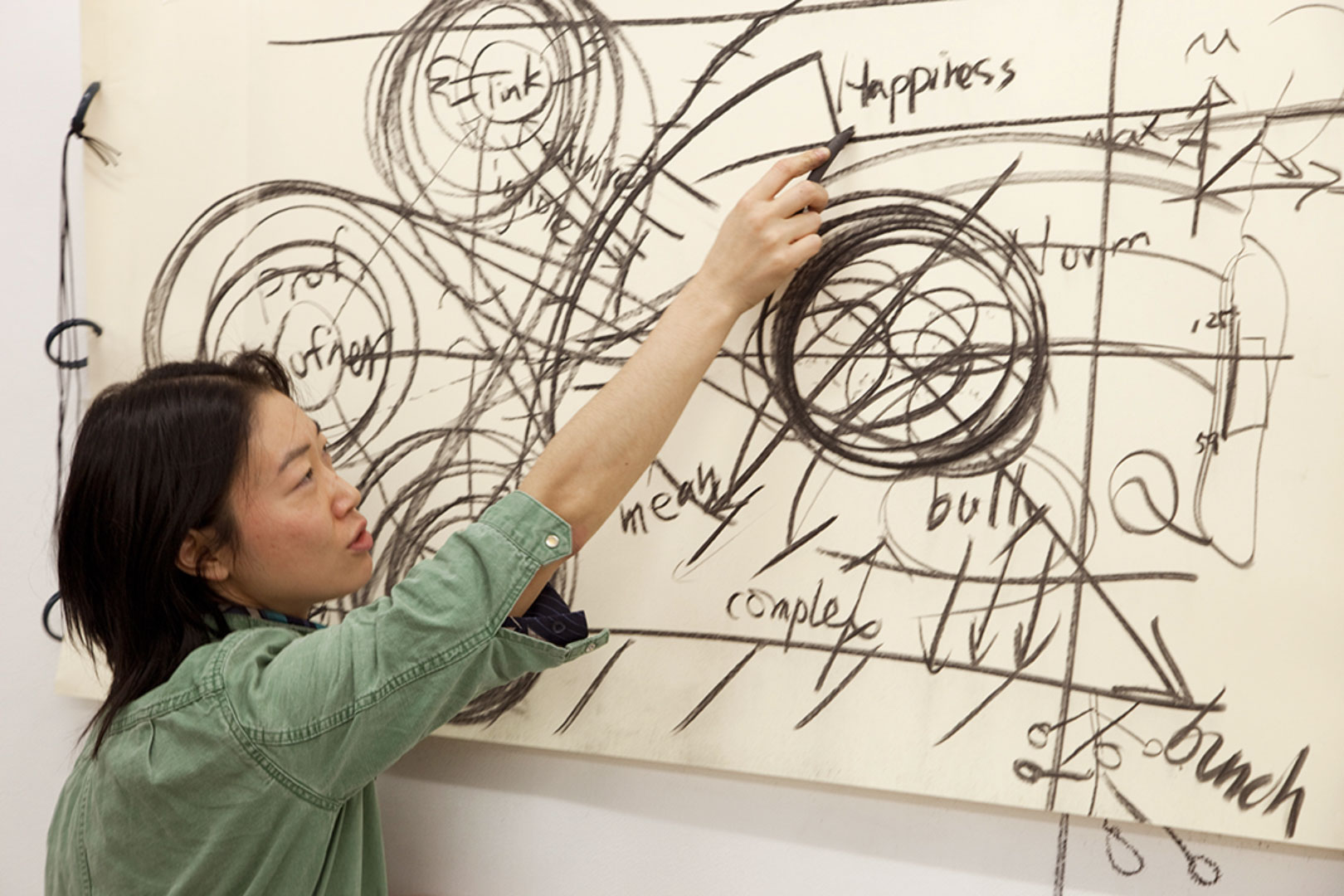
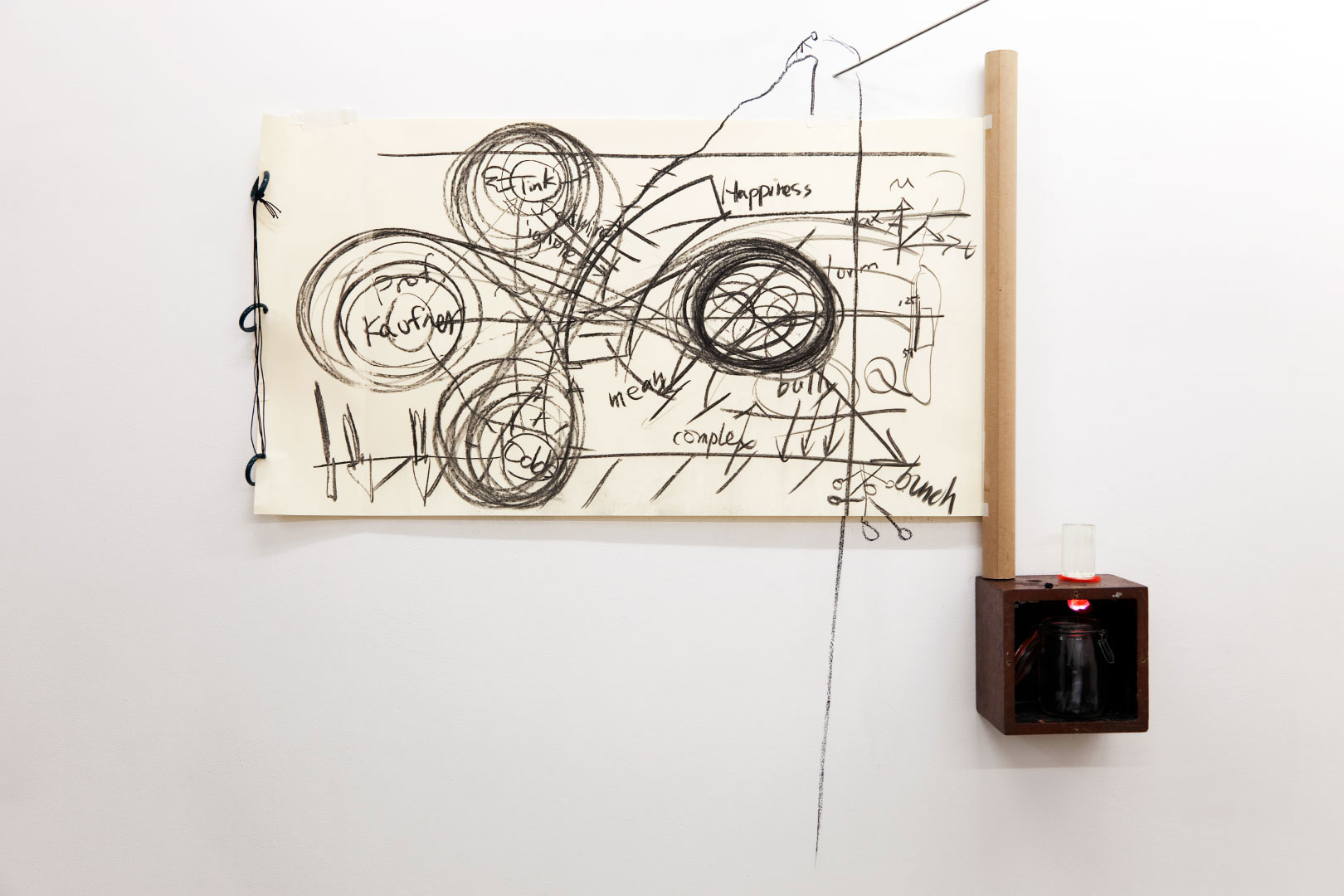
(draw a diagram on a rolled-out paper)
People in this world is divided into four categories. 99.9% of this world is occupied by this type. This type is called Norm, of normal people. 99.9%, so it’s safe to imagine you are in Norm, as well a person next to you. And there’re three other types we can discuss today. It’s always the easiest to start from this type. This type is called Tink, of Tinker Bell, with wings, flying up high by the sky line. Basically Tink is a Madonna type. For example, if Tink is a teacher, she somehow traps all the student at her school, and then all the students come to the class five minutes before the class and do all the homework, preview and even review for exams. They will drive her everywhere. They would even make a lunch box for her. Tink is somebody a Norm admires, but what Norm gets in return is rather sad. She simply ignores Norm. The way to tell who the Tink is in this room is to watch for her friend; the friend that she claims tends to be anorexic or bulimic. You know, likely she has given so much, so much gifts and energy and all these efforts for the Tink, and then nothing, nothing in return.

On the other side, buried in the ground, is this type. This type is called Odds. Odds is buried underground for a long time. A very dark childhood. Her childhood is characterized by a series of bullies from the Norm. Because of that, Odds develops this complex feeling towards normalcies. 99.9% of the world denies her. But there are two interesting things about Odds. Odds is the only kind who spots who the Tink is. Right away. She notices the Tink right away, in 0.01 second, and avoids her. Tink notices Odds back as well. There is a North pole – North pole relationship here, that they kind of repel each other.

And you know, another thing that’s interesting is that Odds is the only one who has a once-in-a-lifetime opportunity to rise above the ground. It’s dramatic, dangerous, she comes out of the ground and sees the sunrise so beautiful, and she gets too excited. Amazing, exciting, all of a sudden, she sees the colors, sees the whole plain, the whole world, the whole ocean and more. She thinks she could go anywhere, everywhere. So she takes this trip, she rides this train that is called, A-train.

A-train! I live in Manhattan up in Harlem. This is Manhattan, and this is the Central Park… What you find when you go to New York is, the subway system sucks. Coming from Japan, it’s really too slow, subway stops at every 5 blocks, and it never really shows me the real deal. This is the time, and this is the velocity, I know the train could run this fast, you know, but somehow the graph kinks down, wheeeen, without showing its real capacity. Why don’t they go up there and stay there? And that’s what A-train does. A-train skips from 59th Street all the way to 125th Street in one go. It skips the whole park. It’s such a great feeling. A-trains’ “A” stands for Acceleration. It feels so good that you almost forget to get off at your destination. Like I may forget to get off at home and it keeps going, and then get to Bronx, and what’s up there? I don’t know. You just keep going and then by the time you realize, you are on top of a mountain in Catskills, standing still. What can you do when you are on the tip of the mountain? The top step of the ladder? This is how all the rock stars commit suicides after their quick success.

Now what she could do instead of killing herself is…
See this opening? Just get off at a station and join the Norm. Or get off at this station and join the Norm. Get off at this station. And by the way, this area is called the Happiness Belt. She was so close. She was so close to Happiness Belt. She could have just lived a whole different life.

(draw circles on papers on two tables)
I’m often wondering what happens when I become the graph myself. Strange Attractor is something that emerges, when you plug into a computer these differential equations. It’s like there are two planes that don’t meet and I as a point would be going around this point. At some point, it starts to lose the gravity and goes to another plane, go around, come back at some point, go around. What’s unpredictable about this point, is the moment it hops between the two circles. Nobody knows how many times it goes around. Maybe it’s once, five times, and two thousand sixty-five times and then, three times, one time, fifty times, two million times… Nobody knows when exactly it’s going to move to another plane.

(go back to the diagram of people)
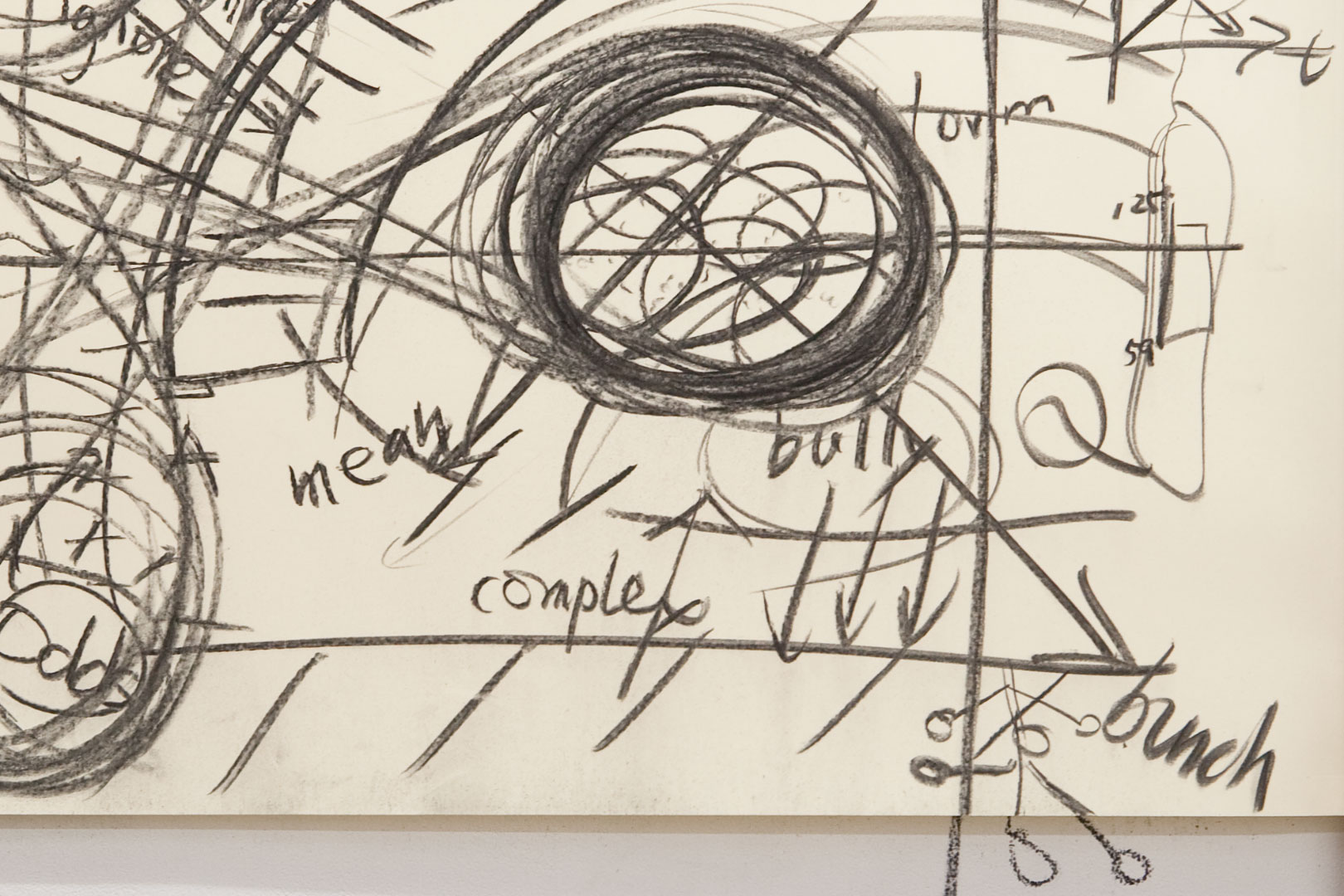
The characteristics of the Norm; they like to form these groups of, I like yellow, pink, or purple, those people who have purple from head to toe. There are people who like boys, girls, who like boys and girls, like theater, like going to yoga on Sundays, whatever that is, Norm tends to gather. This is the type that go to the bathroom together. I call this a bunching effect. This bunching effect increases on this axis, and along this other axis is the level of meanness. So you can imagine what kind of hideous bully has happened down here. Groupie and very evil.

This is how potato grows under the ground. They are all connected, you can’t just get one potato, it always comes with ten, you know. That’s what Norm is. Especially bunchy Norm is in this area. Q-ranked Norm. There are several levels of Norm, and these are the A level Norm, the high level Norm. High level Norm who belongs to the Happiness Belt tends to go over these bridges and interacts with other kinds.

Now, let’s see, opposed to those potatoes, this is how carrots grow. Carrots grow one by one. And that’s this type. This type is called Prof. Kaufner. Prof. Kaufner is a researcher type. She stays in the lab and content with her life and her routines though very lonely. Very solitary, but somehow she is the most interesting one who can speak with Tink. Tink is like a balloon with helium gas and kind of fluffy and real close to this death line. But still below the line, probably because Prof. Kaufner doesn’t admire her and could talk with Tink. And she also accepts Odds. She even researches and writes an essay about a mechanism of A-train. And right here, she is positioned at this bridge to see through the Norm. A very interesting position. A very very interesting position.

The Purpose of this life… the purpose of this life is to find Kaufner.

Do you have any question?
Maybe keep traveling, and one might find Kaufner. Maybe you keep traveling. (offer a donut cushion to an audience)
Oh, you wanna sit on the cushion? This is a …
Ahhhhhhhhhhhhhhhhhhhh!
You may have a hemorrhoid, if someone’s sitting on this type of cushions, there is a hemorrhoid.

(rotate tables with projectors, as live-streamed camera views move along the walls)
It’s very lonely. It’s very lonely to be inside and outside at the same time.

I have to keep traveling, I have to keep travelling to see if I hit somebody.

(go inside the tube and rotate on the industrial spinner)
I wonder what determines the point’s departure to the other side. The traveling point must be so lonely you know. You know you will arrive at the other side, but you don’t know when. What does it feel like when you are travelling to get to other side?

I think the reason why Odds notices Tink right away may be because Tink was an Odds in her last life. And maybe Odds was a Tink. Otherwise it doesn’t make sense because they spot each other right away. The reason why Kaufner sees through everything about the Norm, maybe she used to be…

When you open a math textbook, you notice there are several graphs. At one corner, a graph of x equals 1, and on the other side of the page, when x equals 5. And they’re always separated. It’s always clean. Maybe in real life, the graphs are all super imposed.

And if that’s possible, maybe, maybe I will meet other types. If superimposed, I could possibly get out of this loneliness. I have to keep travelling. I have to keep travelling, and maybe I’ll meet somebody. I will meet somebody if I go to the other side. I wanna see what it feels like to be inside and outside at the same time. My hemorrhoid said,
“It’s very lonely, to be inside and outside at the same time.”
You know, but I had no choice, I had to go to the other side. What does it feel like to know that I have to at some point go back?

(walk to the other corner with the upper body inside the tube)
I just have to get to the other side. I can’t see anybody. Somebody there? Anybody around? It’s lonely. I feel, ahhhhhhhhhhhhhhhh, alone. I don’t know if anybody be at that side. Do you have the same loneliness? Can you somehow get to experience the graph?

(roll on the floor at the corner of the room)
Go away, go away, go away.

Photo: Paula Court , Arturo Vidich , Kei Okano

Video documentation
Take Ninagawa, 2010

Installation

Information

Strange Attractors consists of the careful arrangement of sculpturally altered found objects and recurring performances on the dates that include the numerals 6 or 9, at 16:00. The objects and their placements provide guidance for the artist’s structured improvisation. Sasamoto demonstrates and develops a kaleidoscopic worldview out of personal episodes and a hypothetical mapping of the universe. In an attempt to understand the mathematical concept of strange attractors in dynamical systems, she jumbles her recent obsession for doughnuts, fortunetellers, hemorrhoids, and things detected in the world.

Private Collection in Tokyo

Exhibition History

2010: “Whitney Biennial 2010,” commissioned by The Whitney Biennial, Whitney Museum of American Art, New York
“Strange Attractors,” Take Ninagawa, Tokyo